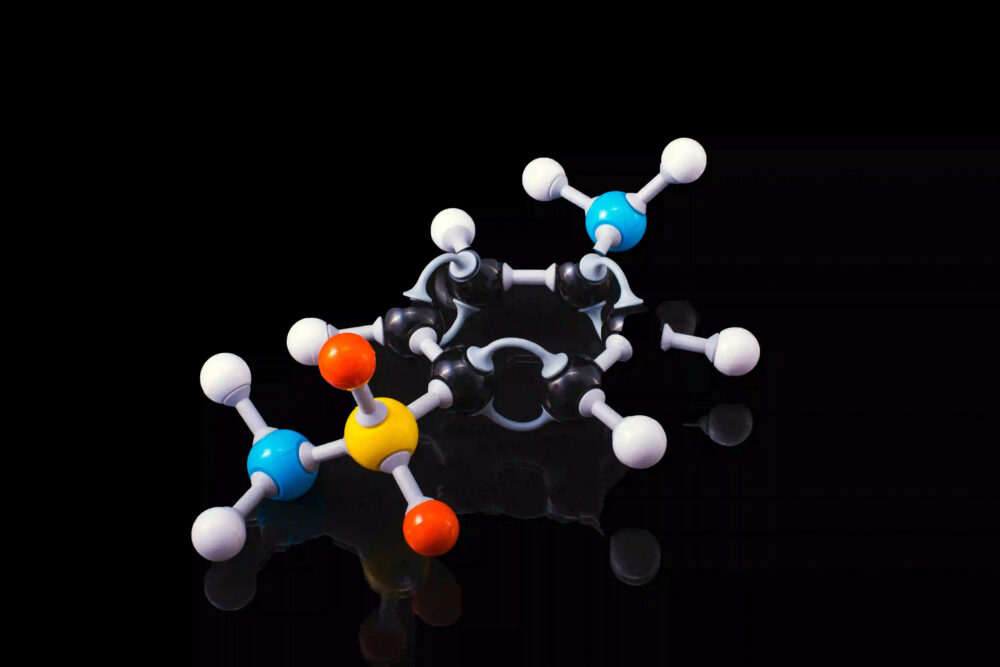
When it comes to unraveling the mysteries of molecular structures, scientists have a powerful tool at their disposal: spectroscopy. Spectroscopy is the study of the interaction between light and matter, and it plays a significant role in various scientific fields, including chemistry, physics, and biology. By utilizing different spectroscopic techniques, researchers can gain valuable insights into the composition, properties, and behavior of molecules. In this article, we will explore the fascinating world of spectroscopy and delve into some key techniques used to probe molecular structures.
Understanding Spectroscopy: The Basics
Before we dive into specific spectroscopy techniques, let’s first understand the fundamental concepts behind this powerful analytical tool. At its core, spectroscopy involves measuring the interaction of light with matter. When light interacts with a molecule, it can be absorbed, transmitted, or scattered, depending on the molecular structure and the characteristics of the incident light.
The interaction between light and matter occurs at specific wavelengths, which correspond to different energy levels within the molecule. By analyzing how light is absorbed or emitted at different wavelengths, scientists can gain valuable information about the molecular structure, chemical bonds, and electronic transitions.
UV-Visible Spectroscopy: Probing Electronic Transitions
UV-visible spectroscopy is a widely used technique for studying the electronic structure of molecules. As the name suggests, this technique involves analyzing the absorption and transmission of ultraviolet (UV) and visible light by a sample. UV-visible spectroscopy provides valuable information about the presence and distribution of electrons within a molecule.
In UV-visible spectroscopy, a beam of light with a range of wavelengths is passed through a sample, and the amount of light absorbed by the sample is measured. Different molecules absorb light at different wavelengths, allowing scientists to identify and characterize specific compounds. This technique is commonly used in fields such as analytical chemistry, biochemistry, and environmental science.
Infrared Spectroscopy: Probing Vibrational Modes
Infrared (IR) spectroscopy is a powerful technique for probing the vibrational modes of molecules. Molecules consist of atoms that are connected by chemical bonds, and these bonds can vibrate in different ways. When infrared light interacts with a molecule, it causes the bonds to stretch, bend, or rotate, resulting in characteristic absorption patterns.
Infrared spectroscopy provides information about the functional groups present in a molecule, helping scientists identify and analyze complex organic compounds. It is widely used in organic chemistry, pharmaceutical research, and forensic analysis. Additionally, IR spectroscopy can be used to study the conformational changes and dynamics of biomolecules like proteins and nucleic acids.
Nuclear Magnetic Resonance (NMR) Spectroscopy: Probing Magnetic Properties
Nuclear Magnetic Resonance (NMR) spectroscopy is a technique that exploits the magnetic properties of atomic nuclei to study molecular structures. NMR spectroscopy is primarily used to determine the connectivity of atoms within a molecule, providing crucial information about molecular structure and conformation.
In NMR spectroscopy, a sample is placed in a strong magnetic field, which aligns the nuclear spins of the atoms within the molecule. By applying radiofrequency pulses, the nuclei can be excited, and their behavior can be observed. The resulting NMR spectrum provides detailed information about the chemical environment, the number of hydrogen atoms, and the connectivity of the atoms within the molecule. NMR spectroscopy is widely used in organic chemistry, medicinal chemistry, and material science.
Raman Spectroscopy: Probing Molecular Vibrations
Raman spectroscopy is a technique that provides information about molecular vibrations and rotational states. Unlike other spectroscopic techniques, Raman spectroscopy measures the scattering of light rather than absorption or transmission. When light interacts with a molecule, it can scatter in different ways, depending on the molecular vibrations and rotational states.
Raman spectroscopy can provide valuable insights into the chemical structure, symmetry, and composition of a sample. It is utilized in various fields, including materials science, pharmaceutical analysis, and environmental monitoring. Raman spectroscopy is particularly useful for non-destructive analysis, as it requires minimal sample preparation and can be performed on solids, liquids, and gases.
Mass Spectrometry: Probing Molecular Mass and Structure
Although not strictly a spectroscopic technique, mass spectrometry plays a crucial role in elucidating molecular structures. Mass spectrometry is a powerful analytical tool used to determine the molecular mass, fragmentation pattern, and structural information of molecules.
In mass spectrometry, a sample is ionized, and the resulting ions are separated based on their mass-to-charge ratio. By measuring the mass-to-charge ratios of the ions, scientists can determine the molecular mass and identify the presence of specific atoms or functional groups. Mass spectrometry is widely used in the fields of organic chemistry, biochemistry, and proteomics.
Expanding Our Understanding of Molecular Structures
Spectroscopy techniques have revolutionized our understanding of molecular structures and their properties. By harnessing the power of light, scientists can probe the intricate details of chemical bonds, electronic transitions, and vibrational modes, paving the way for advances in fields such as materials science, drug discovery, and environmental analysis.
As technology continues to advance, spectroscopy techniques are becoming more powerful, sensitive, and versatile. They are enabling researchers to explore new frontiers, unravel complex molecular interactions, and develop innovative solutions to global challenges.
In conclusion, spectroscopy techniques provide invaluable tools for probing molecular structures with light. Through UV-visible spectroscopy, infrared spectroscopy, NMR spectroscopy, Raman spectroscopy, and mass spectrometry, scientists can unlock the secrets of molecules, unraveling their composition, properties, and behavior. By continually advancing these techniques, scientists are pushing the boundaries of knowledge and ushering in a new era of discovery and innovation.# Physical Chemistry Insights:# Physical Chemistry Insights: Expl# Physical Chemistry Insights: Exploring# Physical Chemistry Insights: Exploring Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics:# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Pe# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Sub# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics:# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quant# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quant# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and sub# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the sub# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newton# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counter# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterint# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle D# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle D# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central ten# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central ten# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-p# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle d# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-p# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle d# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This d# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This d# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encaps# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-s# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Sch# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schr# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrö# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schröd# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two sl# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Sch# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Unc# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schr# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrö# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schröd# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is He# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisen# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determin# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predict# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quant# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wave# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability am# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplit# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observ# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quant# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wave# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics:# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Un# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unr# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unravel# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Stat# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Sch# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schr# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrö# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large en# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schröd# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Expl# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macro# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics:# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Micro# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macro# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Stat# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Bolt# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltz# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Bolt# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltz# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macro# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Bolt# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltz# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Ent# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Bolt# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltz# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Bolt# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltz# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Ent# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Ent# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical En# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Stat# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various en# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common en# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the micro# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Brid# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encaps# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap:# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quant# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical En# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Stat# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical en# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These en# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-E# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein cond# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Sim# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Adv# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Brid# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap:# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quant# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macro# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and re# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quant# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (D# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT),# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion:# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Expl# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Front# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unravel# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilar# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the front# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion:# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Un# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unve# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Myst# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical therm# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By del# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the mysteries# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we gain# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the mysteries of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we gain insights# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the mysteries of molecular# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we gain insights into# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the mysteries of molecular systems# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we gain insights into the# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the mysteries of molecular systems,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we gain insights into the behavior# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the mysteries of molecular systems, one# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we gain insights into the behavior of# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the mysteries of molecular systems, one fascinating# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we gain insights into the behavior of individual# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the mysteries of molecular systems, one fascinating insight# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we gain insights into the behavior of individual particles# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the mysteries of molecular systems, one fascinating insight at# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we gain insights into the behavior of individual particles,# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the mysteries of molecular systems, one fascinating insight at a# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we gain insights into the behavior of individual particles, while# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the mysteries of molecular systems, one fascinating insight at a time# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we gain insights into the behavior of individual particles, while statistical# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a fascinating journey into the heart of physical chemistry, where we delve deep into the realms of quantum mechanics and statistical thermodynamics. In this exploration, we will unravel the mysteries of matter and energy at the molecular level, gaining insights that shape our understanding of the physical world.
Quantum Mechanics: Peering into the Subatomic Realm
Quantum mechanics, often regarded as one of the most profound theories in physics, provides a framework for understanding the behavior of particles at the subatomic level. At this scale, classical Newtonian mechanics no longer apply, and the strange and counterintuitive phenomena of the quantum world emerge.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like behavior. This duality is beautifully encapsulated in the famous double-slit experiment, where particles display interference patterns similar to waves when passed through a barrier with two slits.
Uncertainty Principle
Another cornerstone of quantum mechanics is Heisenberg’s uncertainty principle, which states that the more precisely the position of a particle is known, the less precisely its momentum can be determined, and vice versa. This fundamental principle imposes limits on our ability to simultaneously measure certain pairs of complementary variables, fundamentally altering our classical notions of determinism and predictability.
Quantum States and Operators
In quantum mechanics, physical systems are described by wavefunctions, mathematical functions that encode the probability amplitudes of different quantum states. Operators, representing physical observables such as position, momentum, and energy, act on these wavefunctions to extract meaningful information about the system.
Statistical Thermodynamics: Unraveling the Complexity of Molecular Systems
Statistical thermodynamics provides a powerful framework for understanding the behavior of large ensembles of particles, such as the molecules in a gas or a liquid. By considering the statistical distribution of these particles’ energy states, we can derive macroscopic thermodynamic properties and gain insights into the underlying microscopic dynamics.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the probability distribution of particles over different energy levels in a system at thermal equilibrium. This distribution reveals how energy is distributed among the particles and provides a basis for understanding phenomena such as temperature, entropy, and free energy.
Entropy and the Second Law of Thermodynamics
The concept of entropy, introduced by Ludwig Boltzmann, lies at the heart of the second law of thermodynamics, which states that the entropy of a closed system tends to increase over time. Entropy is a measure of the disorder or randomness of a system, and its increase reflects the tendency of systems to evolve towards states of greater disorder.
Statistical Ensembles
Statistical thermodynamics considers various ensembles of particles, each characterized by different constraints on the system, such as fixed energy, volume, or temperature. The most common ensembles include the microcanonical ensemble, canonical ensemble, and grand canonical ensemble, each providing valuable insights into different aspects of thermodynamic behavior.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected, providing complementary perspectives on the behavior of matter and energy.
Quantum Statistical Mechanics
Quantum statistical mechanics merges the principles of quantum mechanics with those of statistical thermodynamics, offering a unified framework for describing the behavior of molecular systems. This interdisciplinary approach enables us to explore phenomena such as quantum phase transitions, Bose-Einstein condensation, and quantum fluctuations in complex systems.
Molecular Dynamics Simulations
Advances in computational chemistry have facilitated the development of molecular dynamics simulations, which use classical mechanics to simulate the behavior of molecules at the atomic level. By combining quantum mechanical principles with statistical sampling techniques, these simulations provide valuable insights into the dynamic behavior of molecular systems under various conditions.
Quantum Chemistry
Quantum chemistry employs quantum mechanical principles to study the structure, properties, and reactivity of molecules. Techniques such as density functional theory (DFT), coupled cluster theory, and configuration interaction methods enable researchers to accurately predict molecular properties and understand chemical reactions at the quantum level.
Conclusion: Exploring the Frontiers of Physical Chemistry
In conclusion, the realms of quantum mechanics and statistical thermodynamics offer rich and profound insights into the behavior of matter and energy at the molecular level. By combining theoretical frameworks, computational methods, and experimental techniques, researchers continue to push the boundaries of physical chemistry, unraveling the mysteries of the microscopic world and paving the way for new discoveries and innovations.
Join us on this exhilarating journey as we explore the frontiers of physical chemistry, where the strange and fascinating phenomena of quantum mechanics intersect with the complex and intricate dynamics of statistical thermodynamics, shaping our understanding of the fundamental principles that govern the universe.
Let’s embark on this voyage of discovery together, as we unlock the secrets of the quantum world and unravel the mysteries of molecular systems, one fascinating insight at a time.# Physical Chemistry Insights: Exploring Quantum Mechanics and Statistical Thermodynamics
Welcome to a journey through the fascinating realms of physical chemistry, where we delve deep into the fundamental principles governing the behavior of matter and energy at the molecular level. In this exploration, we will unravel the mysteries of quantum mechanics and statistical thermodynamics, two pillars of physical chemistry that provide profound insights into the behavior of atoms and molecules.
Understanding Quantum Mechanics: The Physics of the Small
Quantum mechanics is the branch of physics that describes the behavior of particles at the atomic and subatomic levels. At these tiny scales, classical physics no longer applies, and the principles of quantum mechanics govern the behavior of matter and energy.
Wave-Particle Duality
One of the central tenets of quantum mechanics is the wave-particle duality, which suggests that particles such as electrons and photons exhibit both wave-like and particle-like properties. This duality challenges our classical intuition but provides a powerful framework for understanding the behavior of matter at the atomic scale.
Schrödinger Equation
At the heart of quantum mechanics lies the Schrödinger equation, a fundamental equation that describes the wave function of a quantum system. The solution to this equation provides information about the probability distribution of a particle’s position and momentum, allowing us to make predictions about its behavior.
Quantum States and Energy Levels
In quantum mechanics, particles exist in discrete energy levels, known as quantum states. These energy levels are quantized, meaning they can only take on certain discrete values. Understanding these energy levels is crucial for understanding the behavior of atoms and molecules, as they dictate how particles interact and behave.
Quantum Mechanical Models
Quantum mechanics provides several models for describing the behavior of atoms and molecules. The most well-known of these is the quantum mechanical model of the hydrogen atom, which describes the behavior of electrons in the hydrogen atom using the Schrödinger equation. This model has been incredibly successful in predicting and explaining experimental observations, laying the foundation for our understanding of atomic structure.
Exploring Statistical Thermodynamics: From Microscopic to Macroscopic
Statistical thermodynamics is the branch of physical chemistry that seeks to understand the behavior of large collections of particles, such as atoms and molecules, in terms of their microscopic properties. By applying statistical methods to the principles of thermodynamics, we can make predictions about the macroscopic behavior of systems.
Boltzmann Distribution
Central to statistical thermodynamics is the Boltzmann distribution, which describes the distribution of energy among the particles in a system at thermal equilibrium. This distribution depends on the temperature of the system and provides insights into the most probable configurations of particles.
Entropy and the Second Law of Thermodynamics
The concept of entropy, a measure of the disorder or randomness of a system, plays a crucial role in statistical thermodynamics. The second law of thermodynamics states that the entropy of an isolated system always increases over time, leading to a greater degree of disorder. Understanding entropy allows us to make predictions about the direction of spontaneous processes and the efficiency of energy conversion.
Partition Functions and Thermodynamic Properties
In statistical thermodynamics, the partition function is a key quantity that encapsulates the statistical properties of a system. By calculating the partition function, we can determine various thermodynamic properties, such as the internal energy, entropy, and free energy of a system. These properties provide valuable insights into the stability and behavior of chemical systems.
Statistical Ensembles
Statistical thermodynamics employs various statistical ensembles, such as the canonical ensemble and the grand canonical ensemble, to describe different types of systems. These ensembles allow us to calculate thermodynamic properties under different conditions, providing a comprehensive understanding of the behavior of matter.
Bridging the Gap: Connecting Quantum Mechanics and Statistical Thermodynamics
While quantum mechanics and statistical thermodynamics may seem like distinct branches of physical chemistry, they are intimately connected. Quantum mechanics provides the microscopic framework for understanding the behavior of individual particles, while statistical thermodynamics bridges the gap between the microscopic and macroscopic worlds, allowing us to make predictions about the behavior of large systems.
Quantum Statistical Mechanics
Quantum statistical mechanics is the marriage of quantum mechanics and statistical thermodynamics, combining the principles of both disciplines to describe the behavior of quantum systems at finite temperatures. This field has led to significant advances in our understanding of complex phenomena such as phase transitions, chemical reactions, and the behavior of materials at extreme conditions.
Quantum Mechanical Treatment of Thermodynamic Properties
In recent years, researchers have developed quantum mechanical methods for calculating thermodynamic properties directly from the underlying quantum mechanical equations. These methods, known as quantum Monte Carlo simulations and density functional theory, provide accurate predictions of thermodynamic properties without the need for empirical parameters, opening up new avenues for understanding and predicting the behavior of complex systems.
Conclusion: Unveiling the Mysteries of Matter and Energy
In conclusion, quantum mechanics and statistical thermodynamics provide powerful frameworks for understanding the behavior of matter and energy at the molecular level. By delving into the principles of quantum mechanics, we gain insights into the behavior of individual particles, while statistical thermodynamics allows us to make predictions about the collective behavior of large systems. Together, these disciplines enable us to unravel the mysteries of matter and energy, paving the way for advances in fields such as chemistry, physics, and materials science. As we continue to explore the frontiers of physical chemistry, we unlock new insights into the nature of the universe, enriching our understanding of the world around us.